sierpinski carpet code::Notice that these areas go to 0 as n goes to infinity sierpinski carpet code

sierpinski carpet code

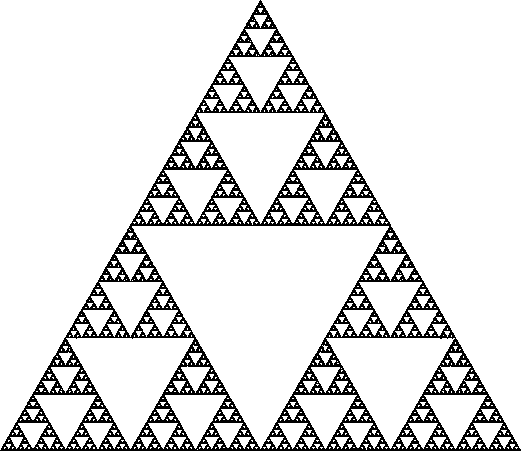
sierpinski carpet code

sierpinski carpet code::Notice that these areas go to 0 as n goes to infinity.
But of course there are many points still left in the carpet.
That is one reason why area is not a useful dimension for this set.
What this basically means is the sierpinski carpet contains a topologically equivalent copy of any compact onedimensional object in the plane.
Thus for any curve contained in the plane, there is a set homeomorphic to the sierpinski carpet that contains the curve.
Mazurkiewicz found an example of a curve which was simultaneously a jordan curve and a cantor curve.
So the sierpinski carpet was actually invented by stefan mazurkiewicz, who in 1913 wrote his ph.
Thesis under the supervision of sierpinski on curves filling a square.
sierpinski carpet code::For curves that cannot be drawn on a 2d surface
without selfintersections, the corresponding universal curve is the , a
higherdimensional generalization sierpinski carpet code